Formula Vector Projection - Symbolab Blog: Advanced Math Solutions - Vector Calculator ... / The two vectors here are the vector to be projected and the vector of the line on which the projection is done.
Formula Vector Projection - Symbolab Blog: Advanced Math Solutions - Vector Calculator ... / The two vectors here are the vector to be projected and the vector of the line on which the projection is done.. Refer to lecture by imperial college london: Vector is a mathematical expression that contains both magnitude and direction. This is a nice compact formula for finding the direction cosines of any vector in $\mathbb{r}^3,$ and it can be generalized to any number of dimensions. How much of one vector goes in the direction of the other vector? the vector projection formula can be written two ways. To do that, we use vector projections.
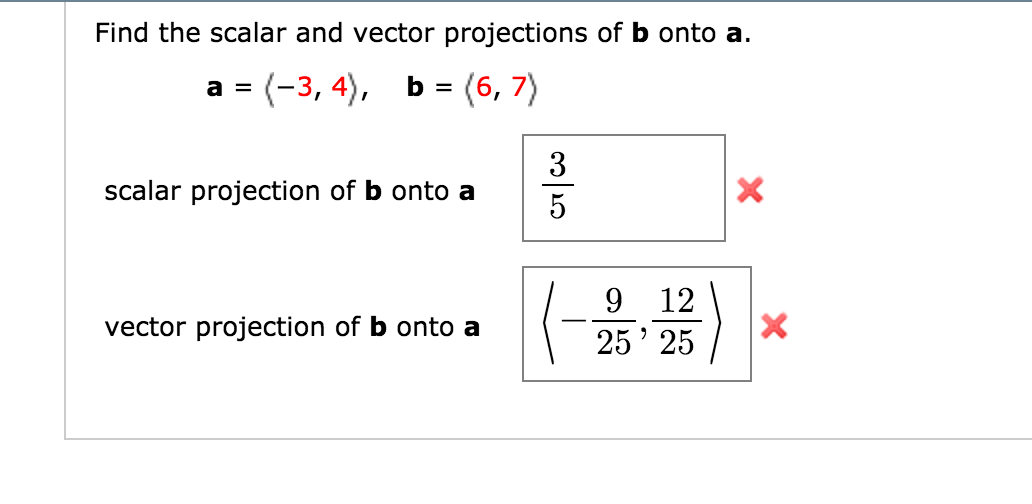
For example, the components along the x and y axes are 3i and 4j. The vector projection of one vector over another is obtained by multiplying the given vector with the cosecant of the angle between the two vectors, and this on further simplification gives the final vector projection formula. The calculator will find the vector projection of one vector onto another, with steps shown. One important use of dot products is in projections. A vector projection of a vector a along some direction is the component of the vector along that direction.
Please watch that video for a nice presentation of the mathematics on this page.
One important use of dot products is in projections. The formula then can be modified as Now let's look at some examples regarding vector projections. Vector is a mathematical expression that contains both magnitude and direction. Download vector projection formula along with the complete list of important formulas used in maths, physics & chemistry. The word projector hints at shadows cast on the wall on which a projector light is shining. The calculator will find the vector projection of one vector onto another, with steps shown. The vector projection of a vector a on (or onto) a nonzero vector b, sometimes denoted. The two vectors here are the vector to be projected and the vector of the line on which the projection is done. To do that, we use vector projections. Refer to lecture by imperial college london: , mark the point on the line at which someone standing on that point could see. I designed this web site and wrote all the mathematical theory, online exercises, formulas and calculators.
To do that, we use vector projections. There are two types of multiplication of vectors. The scalar projection of b onto a is the length of the segment ab shown in the figure below. A simpler way to find out the angle between 2 vectors is the dot product formula. Then the projection of $\vec{bd}$ on that plane can alsow be found in a similar way as shown for the first plane.

Here is the vector projection formula our calculator uses to find the projection of vector a onto the vector b
Projection refer also to khan academy: Introduction of the vector projection formula: For example, the components along the x and y axes are 3i and 4j. The scalar projection of b onto a is the length of the segment ab shown in the figure below. A vector projection of a vector a along some direction is the component of the vector along that direction. This is a nice compact formula for finding the direction cosines of any vector in $\mathbb{r}^3,$ and it can be generalized to any number of dimensions. (also known as the vector component or vector resolution of a in the direction of b). Now let's look at some examples regarding vector projections. Projection of the vector to the axis l is called the scalar, which equals to the length of the segment albl, and the point al is the projection of point a to the in this case, we need to calculate the angle between corresponging vectors, what can be done by using the vectors scalar product formula Componentᵥw = (dot product of v & w) / (w's length). We first consider orthogonal projection onto a line. Refer to lecture by imperial college london: Vector projection whether you are an engineer or an astrologist, you still need to understand how vectors are projected to determine the magnitude as well as the direction of force been applied to any object.
Projecting one vector onto another explicitly answers the question: The version on the left is most simplified, but the version on the right makes the most sense conceptually. For example, the components along the x and y axes are 3i and 4j. To obtain vector projection multiply scalar projection by a unit vector in the direction of the vector onto which the first vector is projected. Now let's look at some examples regarding vector projections.

# for projecting a vector onto another vector.
Projection of the vector to the axis l is called the scalar, which equals to the length of the segment albl, and the point al is the projection of point a to the in this case, we need to calculate the angle between corresponging vectors, what can be done by using the vectors scalar product formula The name is just the same with the names mentioned above: Now let's look at some examples regarding vector projections. The vector projection breaks down a vector like a into components. Vector projection whether you are an engineer or an astrologist, you still need to understand how vectors are projected to determine the magnitude as well as the direction of force been applied to any object. Introduction of the vector projection formula: This is a nice compact formula for finding the direction cosines of any vector in $\mathbb{r}^3,$ and it can be generalized to any number of dimensions. The scalar projection of b onto a is the length of the segment ab shown in the figure below. As v and puv share the same direction, and assuming the v is normalized, puv can be defined as note that this final formula for puv already assumes that v is normalized. # for projecting a vector onto another vector. A vector projection is the idea that the vector (magnitude and direction) can be broken down into component parts along an arbitrarily defined set of dimensional. Componentᵥw = (dot product of v & w) / (w's length). The dot product (inner product).

Komentar
Posting Komentar